Analyzing Math Errors: Conceptual vs. Computation Errors
By Mary Montero
Share This Post:

It’s not secret that I’m a huge fan of error analysis. I have blogged extensively about explicitly teaching error analysis and find that it increases student understanding of individual concepts in incredible ways!
How I Teach Error Analysis
I am often asked how I teach my students to identify errors. Especially when they just don’t know where to begin. Teachers also ask me about teaching students to find errors in not only the tasks I have created, but also in students’ own individual work. Teaching kids to see the difference between a conceptual error and a computational error is the best way that I have found to get students well on their way to analyzing errors!

Although I use this to teach kids to complete error analysis tasks, I also HEAVILY rely on this skill as students work through their own errors. I can have very high-ability students constantly making silly computational mistakes. However, it isn’t until I point this out to them that they finally slow down and stop making them.
Teaching the difference between conceptual and computational mistakes is also a great way to prepare for state testing. Not only will students probably have to do some sort of error analysis on the test, but they also need to examine their work to find errors. It also gives them a great strategy to use if they get an answer that doesn’t match up to any multiple choice answers.
There are two main types of errors that I teach my students to look out for in their own work when completing error analysis tasks. Almost every single error will fall under one of these two types.
Types of Math Errors Students Make
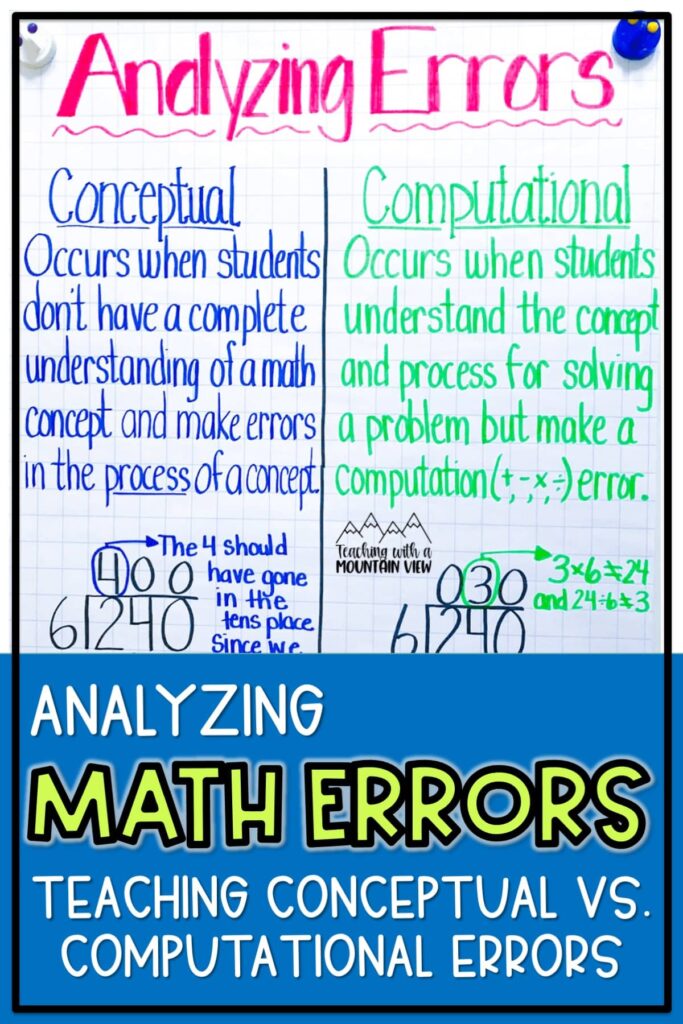
Conceptual Errors
These are errors that students make when they don’t have a complete understanding of the math concepts. So, they end up making errors in the process. These errors are so common when you get into multi-step word problems, multi-digit multiplication, or long division. There are just so many steps in the process of these problems that students tend to have a hard time not missing a single step.
Computational Errors:
Computational errors happen when students understand the concept but make careless errors in computation. These are mistakes made when multiplying, dividing, adding, or subtracting. The process was completed correctly, and the student usually has a solid understanding of the concept, but somewhere along the way, they miscalculated. These may seem like more simple errors, but they can really trip students up. How many of us have ever had a student swear up and down that 6 times 7 is 36? *ME!*
So, how do I teach my students to figure out which type of math error they’ve made?
First
It is very rare that I will tell my students what error they have made in their work. I want to challenge them to figure it out on their own. So, when I see that they have a wrong answer, I ask them to go back and figure out where something went wrong. Because I resist the urge to tell them right away where their error is, my students tend to get a lot more practice identifying them!
Along these same lines, I know that the vast majority of my students are capable of completing a task I assign. This give me confidence that they should, then, also be able to find their mistakes. However, if they aren’t completing it accurately AND they can’t find and fix their own errors, I probably need to rethink whether or not it’s appropriate to be assigning them this type of work to complete independently.
Second
when I introduce a concept, I always, always, always create anchor charts with students and complete interactive notebook activities with them so that they have step-by-step procedures for completing tasks right at their fingertips. I have them go back and reference their notebooks while they are looking at their errors. Usually, they can follow the anchor chart step-by-step to make sure they haven’t made a conceptual error, and if they have, they can identify it.
Third
I let them use a calculator. When worst comes to worst, and they are fairly certain they haven’t made a conceptual mistake to identify, I let them get out a calculator and start computing, step-by-step to see where they’ve made a mistake.
IF, after taking these steps, a student can’t figure out their mistake (especially if I find that it’s a conceptual mistake), I know I need to go back and do some individual reteaching with them because they don’t have a solid understanding of the concept.
This FREE addition error analysis is a good place to start, no matter the grade level. I show them the process of walking through the problem and how best to complete an error analysis task.
If you want to read even more about explicitly incorporating error analysis into your classroom, click the image below!
Mary Montero
I’m so glad you are here. I’m a current gifted and talented teacher in a small town in Colorado, and I’ve been in education since 2009. My passion (other than my family and cookies) is for making teachers’ lives easier and classrooms more engaging.












